The image above represents standard deviation. To calculate the standard deviation, three essential parameters are needed and these parameters are Number of possible outcomes in any single trial (n), Probability of a success in any single trial (p) and Probability of a failure in any single trial (q).
The formula for calculating standard deviation:
σ = √(npq)
Where;
σ = Standard deviation
n = Number of Possible Outcomes in Any Single Trial
p = Probability of a Success in Any Single Trial
q = Probability of a Failure in Any Single Trial
Let’s solve an example;
Find the standard deviation when the number of possible outcomes in any single trial is 14, the probability of a success in any single trial is 0 and the probability of a failure in any single trial is 1.
This implies that;
n = Number of Possible Outcomes in Any Single Trial = 14
p = Probability of a Success in Any Single Trial = 0
q = Probability of a Failure in Any Single Trial = 1
σ = √(npq)
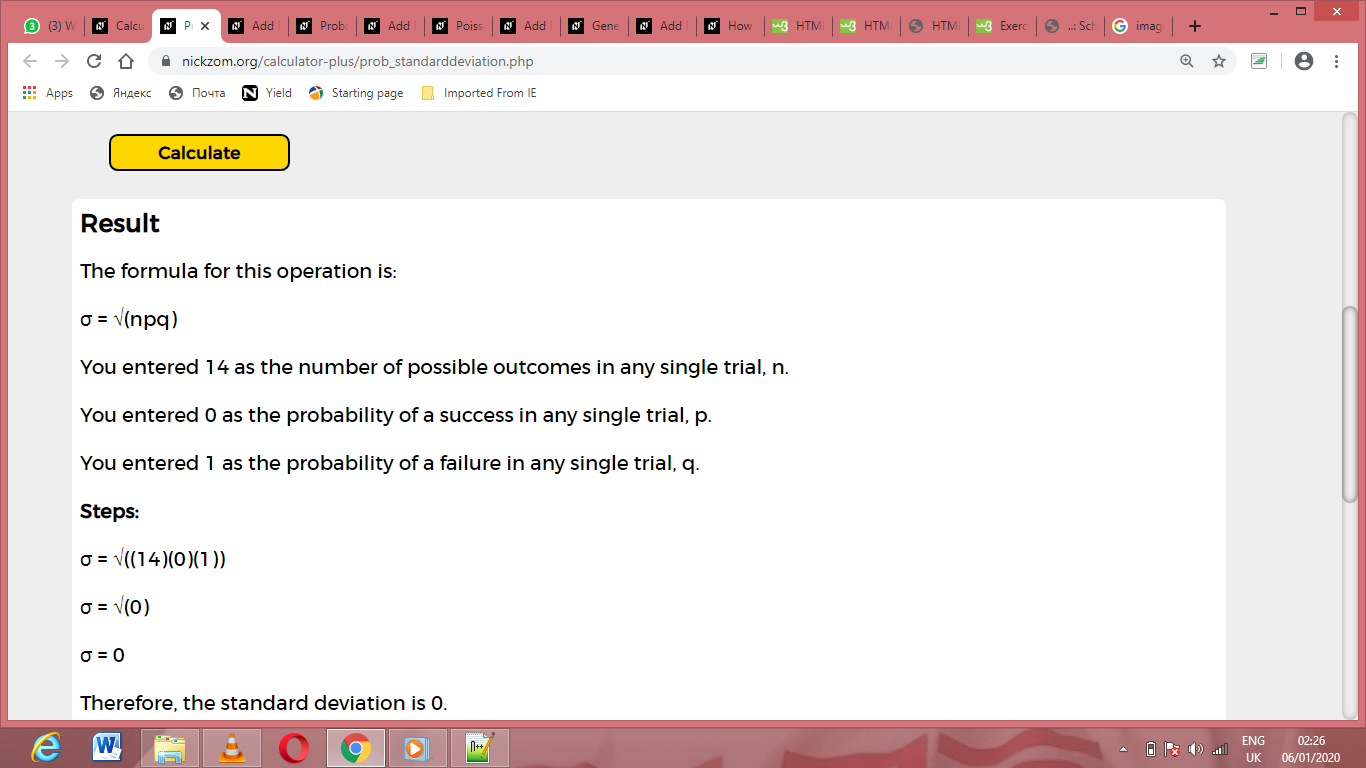
So, σ = √((14)(0)(1))
σ = √(0)
σ = 0
Therefore, the standard deviation is 0.
Calculating the Number of Possible Outcomes in Any Single Trial when the Standard Deviation, the Probability of a Success in Any Single Trial and the Probability of a Failure in Any Single Trial are Given
n = σ2 / pq
Where;
n = Number of Possible Outcomes in Any Single Trial
σ = Standard deviation
p = Probability of a Success in Any Single Trial
q = Probability of a Failure in Any Single Trial
Let’s solve an example;
Given that standard deviation is 5, the probability of a success in any single trial is 1 and the probability of a failure in any single trial is 1. Find the number of possible outcomes in any single trial?
Master Every Calculation Instantly
Unlock solutions for every math, physics, engineering, and chemistry problem with step-by-step clarity. No internet required. Just knowledge at your fingertips, anytime, anywhere.
This implies that;
σ = Standard deviation = 5
p = Probability of a Success in Any Single Trial = 1
q = Probability of a Failure in Any Single Trial = 1
n = σ2 / pq
Then, n = 52 / 1 x 1
n = 25 / 1
n = 25
Therefore, the number of possible outcomes in any single trial is 25.
Read more: How to Calculate and Solve for Mean | Probability
Calculating Probability of a Success in Any Single Trial when the Standard Deviation, the Number of Possible Outcomes in Any Single Trial and the Probability of a Failure in Any Single Trial are Given
p = σ2 / nq
Where;
p = Probability of a Success in Any Single Trial
σ = Standard deviation
n = Number of Possible Outcomes in Any Single Trial
q = Probability of a Failure in Any Single Trial
Let’s solve an example;
Given that standard deviation is 2, the number of possible outcomes in any single trial is 2 and the probability of a failure in any single trial is 1. Find the probability of a success in any single trial?
This implies that;
σ = Standard deviation = 2
n = Number of Possible Outcomes in Any Single Trial = 2
q = Probability of a Failure in Any Single Trial = 1
p = σ2 / nq
Then, p = 22 / 2 x 1
p = 4 / 2
p = 2
Therefore, the probability of a success in any single trial is 2.
Read more: How to Calculate and Solve for Success and Failure | Probability
Calculating Probability of a Failure in Any Single Trial when the Standard Deviation, the Number of Possible Outcomes in Any Single Trial and the Probability of a Success in Any Single Trial are Given
q = σ2 / nq
Where;
q = Probability of a Failure in Any Single Trial
σ = Standard deviation
n = Number of Possible Outcomes in Any Single Trial
p = Probability of a Success in Any Single Trial
Let’s solve an example;
Given that standard deviation is 3, the number of possible outcomes in any single trial is 2, the probability of a success in any single trial is 1. Find the probability of a failure in any single trial?
This implies that;
σ = Standard deviation = 3
n = Number of Possible Outcomes in Any Single Trial = 2
p = Probability of a Success in Any Single Trial = 1
q = σ2 / nq
q = 32 / 2 x 1
That is, q = 9 / 2
q = 4.5
Therefore, the probability of a failure in any single trial is 4.5.
How to Calculate and Solve for Standard Deviation Using Nickzom Calculator
Nickzom Calculator – The Calculator Encyclopedia is capable of calculating the standard deviation.
To get the answer and workings of the standard deviation using the Nickzom Calculator – The Calculator Encyclopedia. First, you need to obtain the app.
You can get this app via any of these means:
Web – https://www.nickzom.org/calculator-plus
To get access to the professional version via web, you need to register and subscribe for NGN 1,500 per annum to have utter access to all functionalities.
You can also try the demo version via https://www.nickzom.org/calculator
Android (Paid) – https://play.google.com/store/apps/details?id=org.nickzom.nickzomcalculator
Android (Free) – https://play.google.com/store/apps/details?id=com.nickzom.nickzomcalculator
Apple (Paid) – https://itunes.apple.com/us/app/nickzom-calculator/id1331162702?mt=8
Once, you have obtained the calculator encyclopedia app, proceed to the Calculator Map, then click on Probability under Mathematics.
Now, Click on Standard Deviation under Probability
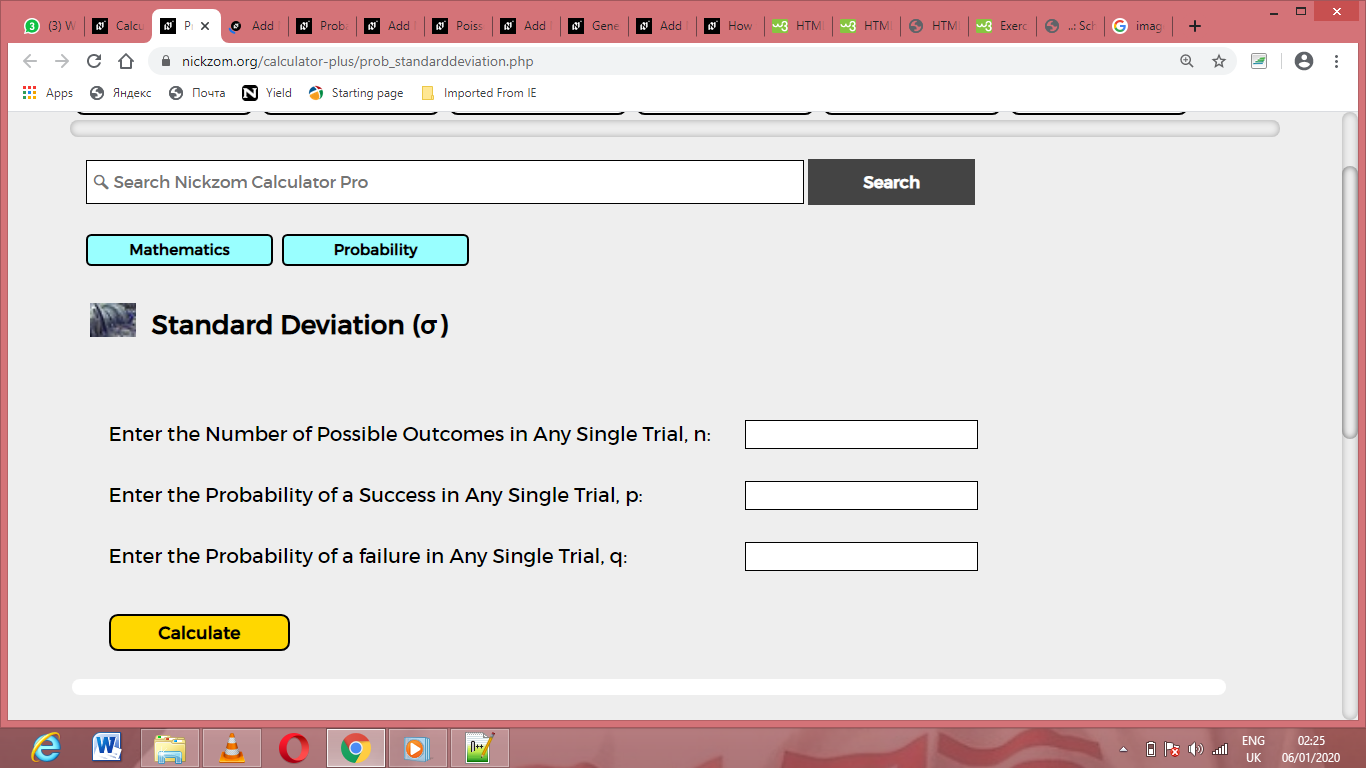
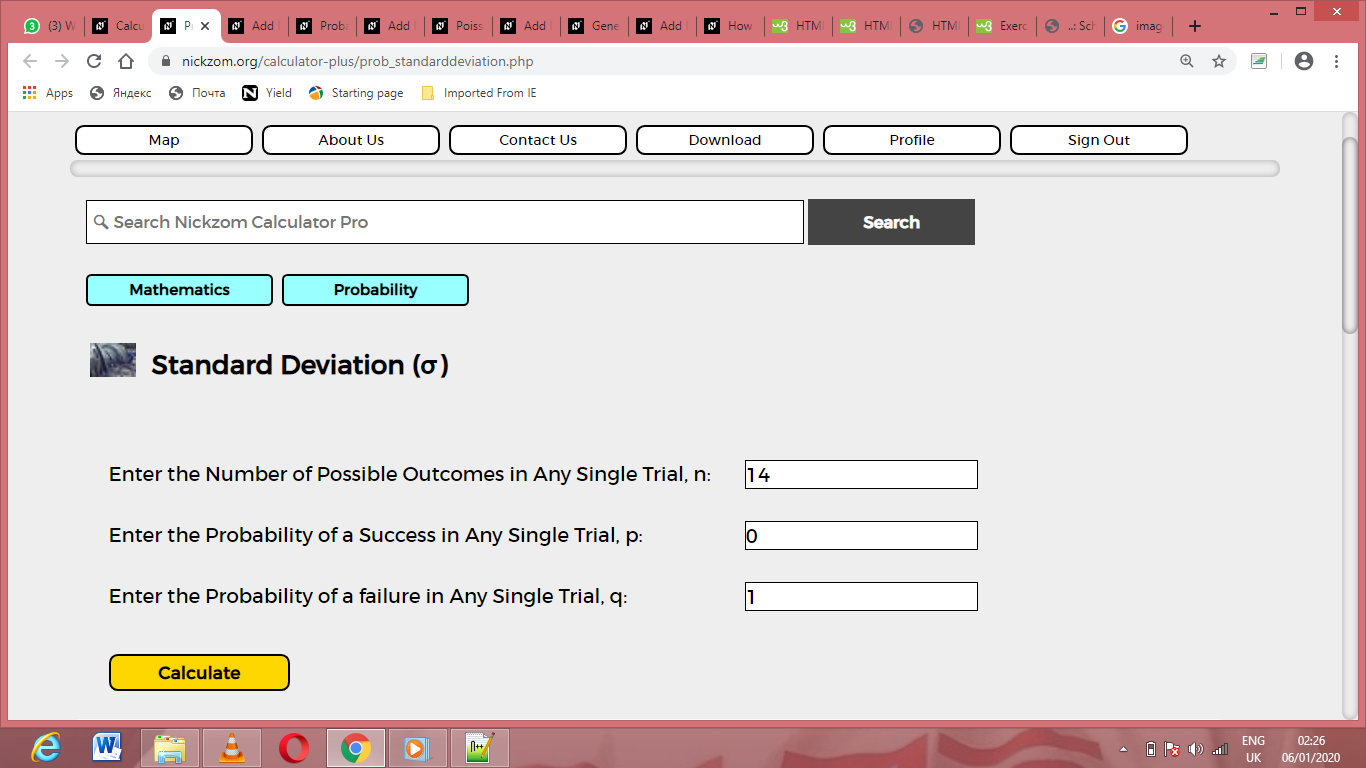
The screenshot below displays the page or activity to enter your values, to get the answer for the standard deviation according to the respective parameters which are the Number of possible outcomes in any single trial (n), Probability of a success in any single trial (p) and Probability of a failure in any single trial (q).
Now, enter the values appropriately and accordingly for the parameters as required by the Number of possible outcomes in any single trial (n) is 14, Probability of a success in any single trial (p) is 0 and Probability of a failure in any single trial (q) is 1.
Finally, Click on Calculate
As you can see from the screenshot above, Nickzom Calculator– The Calculator Encyclopedia solves for the standard deviation and presents the formula, workings and steps too.